One wonders if this doesn’t have IgNobel potential. Of course, it’s not particularly useful, so I doubt it, but it does have that slightly silly appeal :)
Vauxhall Motors commissioned a University of London researchers by the name of Simon Blackburn to figure out how much space any given car needs to parallel park without ‘see-sawing’.
As he says in his paper:
“I want to parallel park, and I’ve found a space. The road is wide, but the space looks narrow. I’m not interested in shuffling back and forth to get into the space: I want to reverse into the space at full lock, and then drive straight forward into the middle of the space to park. How narrow can the space be so that I can do this? This report uses some straightforward geometry to compute the smallest length that the space can be.”
And the final equation, which he came to using some circles and a bit of Pythagorean goodness, looks like this…
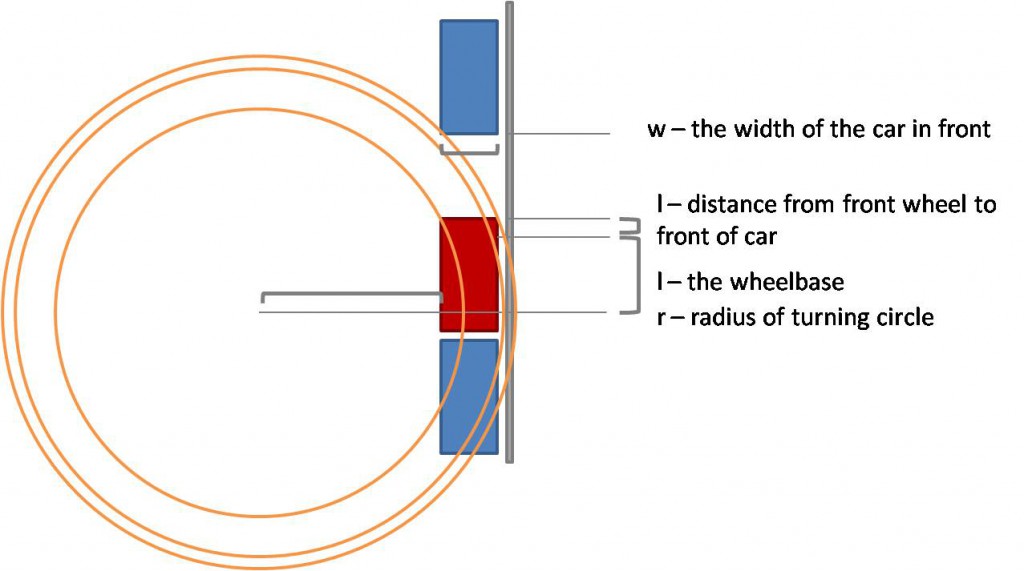
- r is the radius of my car’s turning circle (curb to curb)
- l is my car’s wheelbase, defined as the distance between the centres of the front and rear wheels
- k is the distance from the centre of my front wheel to the front of my car, and
- w is the width of the car in front of mine once I’m done parking
(npr’s got some good graphics here)
So yes, folks, there you have it! Of course, much the same effect can be achieved by practice, and various other techniques, but it’s good to know that, given the time, equipment and facility for maths almost no one has while attempting to parallel park, you could be absolutely certain whether that gap was big enough…Happy parking!
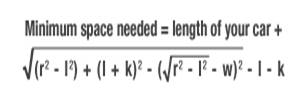
Pingback: Tweets that mention A little bit of fun: how to (mathematically) park your car | misc.ience -- Topsy.com()
Pingback: A Dead Prius Battery Will Cost You Thousands - Or Will It? | Car Extended()
Pingback: Did you see the one about . . . « Homepaddock()